Unlock a world of possibilities! Login now and discover the exclusive benefits awaiting you.
Featured Content
-
How to contact Qlik Support
Qlik offers a wide range of channels to assist you in troubleshooting, answering frequently asked questions, and getting in touch with our technical e... Show MoreQlik offers a wide range of channels to assist you in troubleshooting, answering frequently asked questions, and getting in touch with our technical experts. In this article, we guide you through all available avenues to secure your best possible experience.
For details on our terms and conditions, review the Qlik Support Policy.
Index:
- Support and Professional Services; who to contact when.
- Qlik Support: How to access the support you need
- 1. Qlik Community, Forums & Knowledge Base
- The Knowledge Base
- Blogs
- Our Support programs:
- The Qlik Forums
- Ideation
- How to create a Qlik ID
- 2. Chat
- 3. Qlik Support Case Portal
- Escalate a Support Case
- Phone Numbers
- Resources
Support and Professional Services; who to contact when.
We're happy to help! Here's a breakdown of resources for each type of need.
Support Professional Services (*) Reactively fixes technical issues as well as answers narrowly defined specific questions. Handles administrative issues to keep the product up-to-date and functioning. Proactively accelerates projects, reduces risk, and achieves optimal configurations. Delivers expert help for training, planning, implementation, and performance improvement. - Error messages
- Task crashes
- Latency issues (due to errors or 1-1 mode)
- Performance degradation without config changes
- Specific questions
- Licensing requests
- Bug Report / Hotfixes
- Not functioning as designed or documented
- Software regression
- Deployment Implementation
- Setting up new endpoints
- Performance Tuning
- Architecture design or optimization
- Automation
- Customization
- Environment Migration
- Health Check
- New functionality walkthrough
- Realtime upgrade assistance
(*) reach out to your Account Manager or Customer Success Manager
Qlik Support: How to access the support you need
1. Qlik Community, Forums & Knowledge Base
Your first line of support: https://community.qlik.com/
Looking for content? Type your question into our global search bar:
The Knowledge Base
Leverage the enhanced and continuously updated Knowledge Base to find solutions to your questions and best practice guides. Bookmark this page for quick access!
- Go to the Official Support Articles Knowledge base
- Type your question into our Search Engine
- Need more filters?
- Filter by Product
- Or switch tabs to browse content in the global community, on our Help Site, or even on our Youtube channel
Blogs
Subscribe to maximize your Qlik experience!
The Support Updates Blog
The Support Updates blog delivers important and useful Qlik Support information about end-of-product support, new service releases, and general support topics. (click)The Qlik Design Blog
The Design blog is all about product and Qlik solutions, such as scripting, data modelling, visual design, extensions, best practices, and more! (click)The Product Innovation Blog
By reading the Product Innovation blog, you will learn about what's new across all of the products in our growing Qlik product portfolio. (click)Our Support programs:
Q&A with Qlik
Live sessions with Qlik Experts in which we focus on your questions.Techspert Talks
Techspert Talks is a free webinar to facilitate knowledge sharing held on a monthly basis.Technical Adoption Workshops
Our in depth, hands-on workshops allow new Qlik Cloud Admins to build alongside Qlik Experts.Qlik Fix
Qlik Fix is a series of short video with helpful solutions for Qlik customers and partners.The Qlik Forums
- Quick, convenient, 24/7 availability
- Monitored by Qlik Experts
- New releases publicly announced within Qlik Community forums (click)
- Local language groups available (click)
Ideation
Suggest an idea, and influence the next generation of Qlik features!
Search & Submit Ideas
Ideation GuidelinesHow to create a Qlik ID
Get the full value of the community.
Register a Qlik ID:
- Go to register.myqlik.qlik.com
If you already have an account, please see How To Reset The Password of a Qlik Account for help using your existing account. - You must enter your company name exactly as it appears on your license or there will be significant delays in getting access.
- You will receive a system-generated email with an activation link for your new account. NOTE, this link will expire after 24 hours.
If you need additional details, see: Additional guidance on registering for a Qlik account
If you encounter problems with your Qlik ID, contact us through Live Chat!
2. Chat
Incidents are supported through our Chat, by clicking Chat Now on any Support Page across Qlik Community.
To raise a new issue, all you need to do is chat with us. With this, we can:
- Answer common questions instantly through our chatbot
- Have a live agent troubleshoot in real time
- With items that will take further investigating, we will create a case on your behalf with step-by-step intake questions.
3. Qlik Support Case Portal
Log in to manage and track your active cases in the Case Portal. (click)
Please note: to create a new case, it is easiest to do so via our chat (see above). Our chat will log your case through a series of guided intake questions.
Your advantages:
- Self-service access to all incidents so that you can track progress
- Option to upload documentation and troubleshooting files
- Option to include additional stakeholders and watchers to view active cases
- Follow-up conversations
When creating a case, you will be prompted to enter problem type and issue level. Definitions shared below:
Problem Type
Select Account Related for issues with your account, licenses, downloads, or payment.
Select Product Related for technical issues with Qlik products and platforms.
Priority
If your issue is account related, you will be asked to select a Priority level:
Select Medium/Low if the system is accessible, but there are some functional limitations that are not critical in the daily operation.
Select High if there are significant impacts on normal work or performance.
Select Urgent if there are major impacts on business-critical work or performance.
Severity
If your issue is product related, you will be asked to select a Severity level:
Severity 1: Qlik production software is down or not available, but not because of scheduled maintenance and/or upgrades.
Severity 2: Major functionality is not working in accordance with the technical specifications in documentation or significant performance degradation is experienced so that critical business operations cannot be performed.
Severity 3: Any error that is not Severity 1 Error or Severity 2 Issue. For more information, visit our Qlik Support Policy.
Escalate a Support Case
If you require a support case escalation, you have two options:
- Request to escalate within the case, mentioning the business reasons.
To escalate a support incident successfully, mention your intention to escalate in the open support case. This will begin the escalation process. - Contact your Regional Support Manager
If more attention is required, contact your regional support manager. You can find a full list of regional support managers in the How to escalate a support case article.
Phone Numbers
When other Support Channels are down for maintenance, please contact us via phone for high severity production-down concerns.
- Qlik Data Analytics: 1-877-754-5843
- Qlik Data Integration: 1-781-730-4060
- Talend AMER Region: 1-800-810-3065
- Talend UK Region: 44-800-098-8473
- Talend APAC Region: 65-800-492-2269
Resources
A collection of useful links.
Qlik Cloud Status Page
Keep up to date with Qlik Cloud's status.
Support Policy
Review our Service Level Agreements and License Agreements.
Live Chat and Case Portal
Your one stop to contact us.
Recent Documents
-
[SOURCE_UNLOAD ]E: Data error found: No delimiter found after a valid value o...
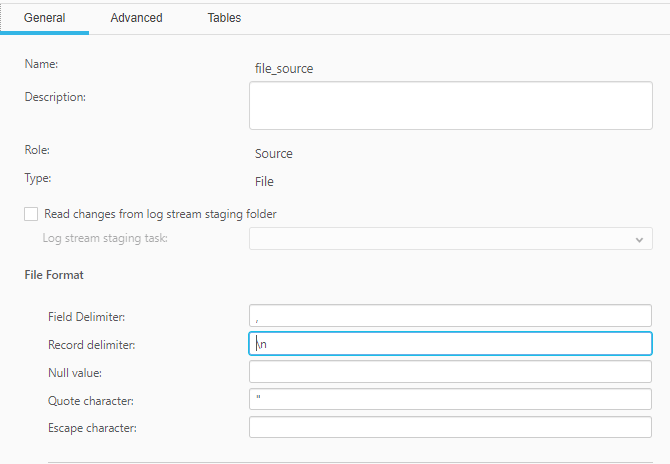
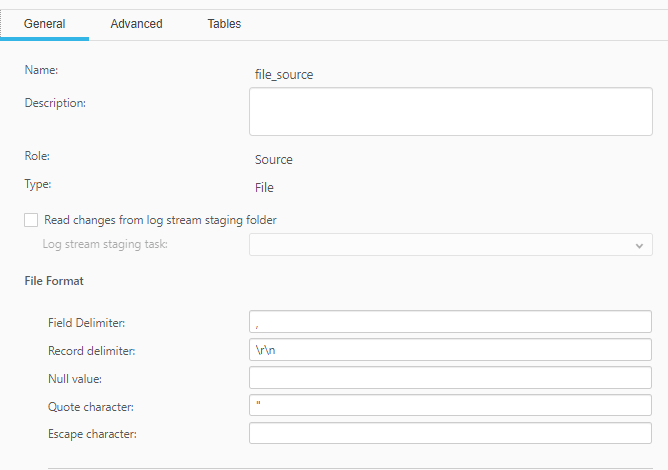
I encountered this error when testing a csv file source endpoint. When I setting up the task, I used the defaults in the Source File endpoint. From l... Show MoreI encountered this error when testing a csv file source endpoint.
When I setting up the task, I used the defaults in the Source File endpoint.
From logs I saw:
00041100: 2022-03-25T10:26:45 [SOURCE_UNLOAD ]E: Data error found: No delimiter found after a valid value on quotes mode - file: C:\tmp\datatest.csv, Record number: 2, Offset: 10 [1020417] (csv_util.c:545)
00041100: 2022-03-25T10:26:45 [SOURCE_UNLOAD ]E: CSV Util failed to write value to buffer [1020417] (csv_util.c:1003)
00041100: 2022-03-25T10:26:45 [SOURCE_UNLOAD ]E: Failed to read record number: 2. [1020417] (file_unload.c:259)
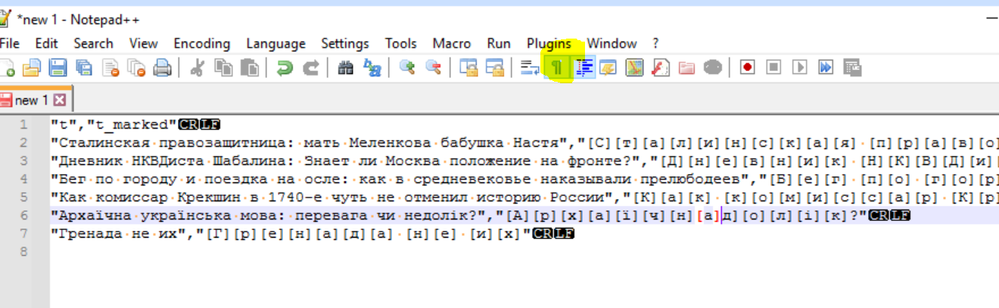
00041100: 2022-03-25T10:26:45 [SOURCE_UNLOAD ]E: Failed to init unloading table. [1020417] (file_unload.c:395)When I opened in Notepad, I saw my csv file had CRFL carriage return and not FL.
I updated the source endpoint settings to resolve the 'Record delimiter field'.
Table was then able to load in the task.
Environment
The information in this article is provided as-is and to be used at own discretion. Depending on tool(s) used, customization(s), and/or other factors ongoing support on the solution below may not be provided by Qlik Support.
-
How to download Qlik Products
Are you looking to download your purchased Qlik Products or download a Trial? All supported on-premise Qlik Products can be downloaded from Qlik's ... Show MoreAre you looking to download your purchased Qlik Products or download a Trial? All supported on-premise Qlik Products can be downloaded from Qlik's Product Download Site.
To access the Download Site, you need an active QlikID. You will be able to see all products your account is eligible for.
Qlik's download are hosted on GitHub. If you are unable to access GitHub and do not have the possibility of enabling exceptions, start a chat with us and we will be able to help you.
You can access the Download Page directly here, or navigate to it from the Community Home page:
- Open the Qlik Community
- Click on Support
- Select Product Downloads
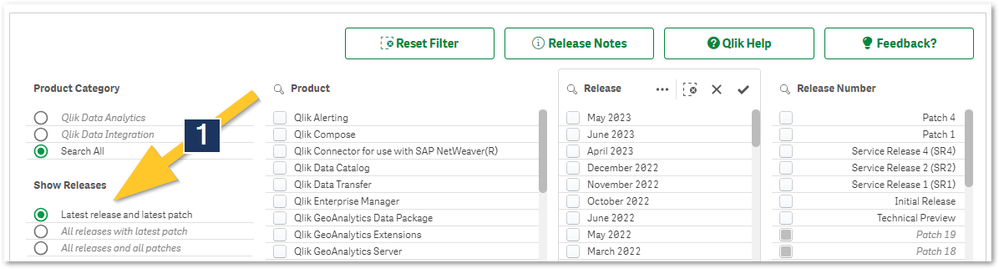
This shows the download page with the Latest release and patch preselected.
- Choose a different filter in (1) Show Releases if you are looking for older product versions. By default, only the Latest release and patch will be displayed.
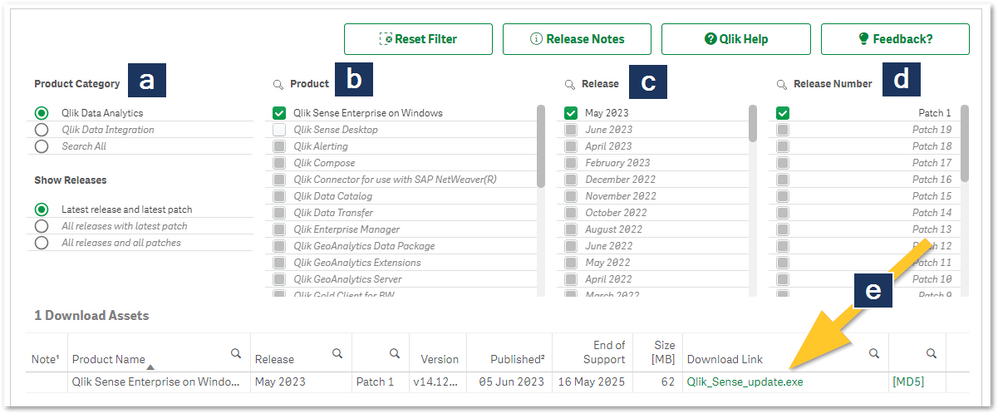
- From here:
- choose your Product Category (Qlik Data Analytics, Qlik Data Integration, Search All),
- your Product,
- your Release,
- and Release Number.
- Then click the Download Link
Unsupported versions are not available for download. See Product Lifecycle for details on what versions have reached end of support.
Alternatively, instead of selecting your product directly, you can also search all available columns:
- Click the Magnifying Glass
- Begin typing your search
- Select the result
- Confirm the result
If you encounter issues with the download site start a chat with us and we will be able to help you right away.
Click here for video transcript
-
No Hub or QMC access after upgrade to Sense November 2017 or higher - recursive ...
Trying to access Qlik Sense Hub or Management Console (QMC) leads to the getting "refused to connect" on the web browser. Investigating the issue with... Show MoreTrying to access Qlik Sense Hub or Management Console (QMC) leads to the getting "refused to connect" on the web browser.
Investigating the issue with the command netstat | findstr :443 confirms there is not service listening on port 443 (or port QMC/Hub proxy is set to listen on).
Case 1:An inspection of the System_Repository log located in C:\ProgramData\Qlik\Sense\Log\Repository\Trace shows an error message relating to being unable to remove soft deleted records. Sample:
Fatal exception Connection must be opened.??Connection must be opened.??Failed to remove soft deleted records??An exception was thrown while invoking the constructor 'Void .ctor()' on type 'DatabaseContext'
Case 2:The Repository Service to update fails on a table that iolates foreign key constraint.
C:\ProgramData\Qlik\Sense\Log\Repository\Trace\QLIKSERVER1_System_Repository
Fatal exception update or delete on table "CompositeEvents" violates foreign key constraint "FK_CompositeEventTimeConstraints_CompositeEvents_ID" on table "CompositeEventTimeConstraints"??Failed to execute query: 'DELETE FROM "CompositeEvents" WHERE "Deleted" = true'. Exception: update or delete on table "CompositeEvents" violates foreign key constraint
Environment:Cause:
In November 2017, February 2018, April 2018 and June 2018, when the Qlik Sense Repository Service initializes, it attempts to clean up the underlying Repository Database. If there are records which have broken referential integrity then the Repository Service will fail to initialize. This is a consequence of a change in behavior in November 2017/February 2018 where the Repository no longer does soft deletes.
This script can be obtained from the official Qlik site: Failed to remove soft deleted records.
If logged on to our support site, it can be directly downloaded from the attachment. The attachment is unavailable for anonymous users.Resolution:
This article uses the recursive (recurse) cleanup sql script. After upgrading to Qlik Sense November 2017 or February 2018, the administrator is unable to access the Hub and/or QMC.
This article also fixes database issues and soft deletes, which cause numerous other problems besides being unable to access the QMC or HUB after an upgrade. This script is necessary to be run if upgrading from an older version to 11.14.3 or newer.
- Stop all services but the Qlik Sense Repository Database
- If using a stand-alone PostgreSQL server to host Qlik Sense content, then stop all Qlik Sense Services
- Take a backup of the Qlik Sense Repository Database
- Sample command using cmd.exe: cd / & mkdir QSR & "C:\Program Files\Qlik\Sense\Repository\PostgreSQL\9.6\bin\pg_dump.exe" -h localhost -p 4432 -U postgres -b -F t -f "c:\QSR\QSR_backup_%date:~-4,4%%date:~-10,2%%date:~-7,2%.tar" QSR
- This assumes that:
- Qlik Sense is installed to C:\Program Files
- That the site is not using a stand-alone PostgreSQL server
- Make any necessary adjustments if neither of those assumptions are true
- This assumes that:
- Sample command using cmd.exe: cd / & mkdir QSR & "C:\Program Files\Qlik\Sense\Repository\PostgreSQL\9.6\bin\pg_dump.exe" -h localhost -p 4432 -U postgres -b -F t -f "c:\QSR\QSR_backup_%date:~-4,4%%date:~-10,2%%date:~-7,2%.tar" QSR
- Download the attached .sql file and place it into the same directory as pg_dump.exe
- Run the following command in cmd.exe (run them one at a time and hit Enter then continue with the next one):
- cd C:\"Program Files"\Qlik\Sense\Repository\PostgreSQL\9.6\bin
- SET PGCLIENTENCODING=utf-8
- chcp 65001
- psql.exe -h localhost -p 4432 -U postgres -d QSR -e -f recurse_cleanup.sql
- Note: If Qlik Sense is installed to a non-default directory then make the necessary adjustments
- Start all Qlik Sense Services
- Validate that the error about Failed to remove soft deleted records is not present in the System_Repository log
- If this does not resolve the issue then do contact Qlik Support for a more customized query
- Stop all services but the Qlik Sense Repository Database
-
Qlik NPrinting task run but do not progress: stuck on same percentage
Qlik NPrinting tasks appear to run but are stuck on the same percentage. Qlik NPrinting can work for days or even weeks, completing tasks correctly. S... Show MoreQlik NPrinting tasks appear to run but are stuck on the same percentage.
Qlik NPrinting can work for days or even weeks, completing tasks correctly. Suddenly, all task stops at different levels of execution. New tasks start, but stay on 0%.
Idle sessions have already been removed by applying How to remove the Idle Connections from Nprinting Related processes.
This error message is shown in the rabbit logs in C:\ProgramData\NPrinting\RabbitMQ for a default installation:
{{badmatch,{error,eacces}}
Qlik NPrinting is installed on a Windows 2019 server, and the version of Qlik NPrinting works with RabbitMQ 4 or higher (see the system requirements page to identify your Qlik NPrinting track).
Environment
- Qlik NPrinting all versions
Resolution
Restarting all Qlik NPrinting and Qlik Sense services can temporarily resolve the problem.
It is possible to mitigate the problem by installing the latest release of Qlik NPrinting, but this is still not a full solution, as the same issue may repeat due to a defect in Windows Server 2019.
A complete solution is to upgrade the operating system where Qlik NPrinting is installed to a higher version.
Cause
The problem is caused by specific updates affecting Windows file system components in Windows Server 2019 that occasionally prevent system access for our messaging service.
-
Force Qlik Replicate to connect to secondary read-only node during Full Load
Instead of pulling the data from the Primary node, which can potentially negatively affect performance, we can force Qlik Replicate to connect to a se... Show MoreInstead of pulling the data from the Primary node, which can potentially negatively affect performance, we can force Qlik Replicate to connect to a secondary read-only node during Full Load.
To do so:
- When starting the task for the first time, Qlik Replicate will connect to the primary database and create a publication with all required articles. Once the creation of the publication is finished and the articles are added to the publication, stop the task.
- Set a new Internal Parameter in the SQL server endpoint, routing the connections to the secondary node.
Parameter: additionalConnectionProperties
Value: ApplicationIntent=ReadOnly
For more information regarding Internal Parameters, see Qlik Replicate: How to set Internal Parameters and what are they for. - Start the reload. As soon as you finished the Full load, stop the task and remove the additional parameter which you added in Step 2.
- Resume the task. All connections will now resume on the primary node.
Environment
- When starting the task for the first time, Qlik Replicate will connect to the primary database and create a publication with all required articles. Once the creation of the publication is finished and the articles are added to the publication, stop the task.
-
Search Folder Growing
Qlik Sense Search Folder is growing in size.Smart search is the global search tool in Qlik Sense, enabling you to search the entire data set in your a... Show MoreQlik Sense Search Folder is growing in size.
Smart search is the global search tool in Qlik Sense, enabling you to search the entire data set in your app from any sheet in the app. See Using Smart Search on the official Qlik Online Help for details.
This search is indexed to disk at %Share%/Apps/Search.
As time goes on, the index grows and commits more disk space.
Environment:
Resolution:
The contents of the Qlik Sense Search folders can be deleted to free up disk space. The content pertains to App ID's, so it would be best to remove those which are not often used. The only effect this would have is increasing the amount of time needed to complete a Smart Search for this same App in the future.
Qlik Sense can also be configured to purge the Search Folder on a schedule. The setting will need to be applied in the settings.ini of either the Qlik Sense Enterprise Server or the Qlik Sense Desktop. For information on how to edit the settings.ini file, reference How to modify Qlik Sense Engine's Settings.ini.
Qlik Sense Server %PROGRAMDATA%/Qlik/Sense/Engine/Settings.ini Qlik Sense Desktop %USERPROFILE%/Documents/Qlik/Sense/Settings.ini Name
ServerPurgeSearchIndexFileInterval
Default Value
3600
Description
- -1 the search index folder is never purged.
- 0 or negative (except -1), the purge happens constantly.
- strictly positive value, the search index folder is purged each ServerPurgeSearchIndexFileInterval seconds.
The purge system removes the unused search index files. In addition, it removes the oldest used search index files until the search index folder is smaller than ServerSearchIndexFileDirectoryMaximumSizeInMBytes.
Name
ServerSearchIndexFileDirectoryMaximumSizeInMBytes
Default Value
-1
Description
Maximum size of the search index directory in megabytes. During the periodic purge, old indexes are removed until the total size of index files is below this threshold. If negative, do not limit the size of the directory.
Make sure to add both parameters mentioned above into the settings.ini, the cleaning will happen at the specified intervall but only after the size limit is reached.
-
Qlik Sense Enterprise on Windows May 2025: Slow Data Load Editor
The Data Load Editor in Qlik Sense Enterprise on Windows 2025 experiences noticeable performance issues. Resolution The issue is caused by defect SU... Show MoreThe Data Load Editor in Qlik Sense Enterprise on Windows 2025 experiences noticeable performance issues.
Resolution
The issue is caused by defect SUPPORT-6006. Qlik is actively working on a fix.
Fix Version
A fix is planned for the next possible patches. Review the Release Notes for SUPPORT-6006.
Workaround
A workaround is available. It is viable as long as the Qlik SAP Connector is not in use.
- In a Windows file browser, navigate to C:\Program Files\Common Files\Qlik\Custom Data\
- Move the QvSapConnectorPackage directory to a different location
No service restart is required.
Internal Investigation ID(s)
SUPPORT-6006
Environment
- Qlik Sense Enterprise on Windows
-
Qlik Replicate: Best Practices and Recovery Steps for Redo Log Retention and Usa...
This article outlines recommended retention policies for Oracle redo logs and provides detailed recovery steps in scenarios where redo logs are needed... Show MoreThis article outlines recommended retention policies for Oracle redo logs and provides detailed recovery steps in scenarios where redo logs are needed. It includes guidance on preserving logs, identifying stream positions, verifying log availability, and using SCN-based recovery with SQL_REDO extraction for issue resolution.
Resolution
Generally, Qlik recommends a 24-hour retention period for redo logs, but this can vary depending on the storage capacity and backup policies of each organization, as they have different requirements.
When customers back up their redo logs, they typically retain backups for at least 2 weeks to 1 month. This allows them to restore the logs if necessary. The specific policy regarding the retention of redo logs in backup media should be clarified with the customer.
Steps to Take When Redo Logs Are Required for Recovery
- Preserve the Redo Logs: When an incident occurs, it’s critical to preserve the redo logs as quickly as possible. This ensures that they can be replayed if necessary.
- Identify the Stream Position: First, identify the stream position of the record that is failing. You can use the task log to locate the problematic record and its corresponding stream position.
- Verify Redo Log Existence: Once you have the stream position, determine the corresponding redo log sequence number and thread number. Verify whether the redo log still exists on disk.
If it exists on disk, copy it to a different filesystem to ensure it is not deleted by RMAN backups.
If the redo log has already been deleted, you will need to restore it from backup media. The DBA can assist in restoring the file from backup.
Replay the Redo Logs
- Locate the Redo Log File: Once the redo log is available, query the v$archived_log view to find where the redo log file is located. You can use the following SQL:
SELECT name, sequence#, thread#, first_change#
FROM v$archived_log
WHERE sequence# = [sequence#] AND thread# = [thread#]; - Get the SCN (System Change Number): Use the first_change# value from the query above as the starting point for the redo log recovery.
- Create a New Task in Replication: In your replication setup, create a new task and add the affected table. In the advanced options, provide the SCN value from the first_change# column, which marks the point where the task should start.
- Verify Reproduction: After setting the SCN, verify whether you can reproduce the issue. If you can, proceed with the next step.
- Log Mining and SQL_REDO: Use standard log mining to extract the SQL_REDO statements from the redo logs. These can be found in the V$logmnr_contents view. The SQL_REDO statements will provide the actual SQL that was executed, which will allow you to check if all column data was included in the redo log.
By following these steps, you can ensure proper recovery from redo logs and address any issues related to missing or inconsistent data.
Environment
- Qlik Replicate
- Preserve the Redo Logs: When an incident occurs, it’s critical to preserve the redo logs as quickly as possible. This ensures that they can be replayed if necessary.
-
Qlik Talend Cloud: Custom Context values lost during CICD artifact redeployment ...
During a CICD artifact update or redeployment, the custom context variables are lost. Cause CICD only publishes a new version of the artifact to the... Show MoreDuring a CICD artifact update or redeployment, the custom context variables are lost.
Cause
CICD only publishes a new version of the artifact to the Qlik Talend Management Console. When creating a task via the Qlik Talend Management Console API call, if the user includes the following parameters in their JSON request (as demonstrated in the Resolution section), it will result in updating the task with the newly published artifact and the default artifact context values.
Resolution
When creating a task via the Qlik Talend Management Console API for the first time, please ensure that the "overrideWithDefaultParameters" parameter is set to false in the "autoUpgradeInfo" section of the JSON body. For example:
"autoUpgradeInfo": {
"autoUpgradable": true,
"overrideWithDefaultParameters": false
}By setting overrideWithDefaultParameters to false during task creation, you can prevent the task from being updated with default context values for future artifact deployments via CICD.
Related Content
Environment
- Qlik Talend Studio
-
Qlik Sense Enterprise on Windows: Create and Sync a UDC with Microsoft Excel Dri...
This article is provided as is. For further customization or assistance, reach out to Qlik's Professional Services or engage in our Qlik Sense Adminis... Show MoreThis article is provided as is. For further customization or assistance, reach out to Qlik's Professional Services or engage in our Qlik Sense Administration and Configuration forum.
This details the steps required to use a Microsoft Excel (XLS) file as a User Directory in the User Directory Connector (UDC).
The instructions in this article are written for Microsoft Office 2016, which has reached end of life as of October 2025. As an alternative, use Microsoft 365 Access Runtime. See Download and install Microsoft 365 Access Runtime.
The required Microsoft Excel Driver may not be installed by default.
- Verify if the drivers are already installed on your server:
Open the Windows Registry Editor and navigate to Computer\HKEY_LOCAL_MACHINE\SOFTWARE\ODBC\
If it is not installed, download the latest Microsoft package.
This file can be directly sourced from the Microsoft Download site. The 64-bit version is required.
Example: Microsoft Access Database Engine 2016 Redistributable - Run the installer
- Refresh the registry editor and verify that the drivers are correctly installed
- Create an XLS file that contains your users
A sample XLS file is attached to this article:
- This file contains two sheets, "MyUsers" and "Attributes"; they can be renamed to fit your naming convention
- The first sheet contains two columns: "userid" and "name"; those names are important and mandatory.
Do not change them, or the import will fail.
- The second sheet contains three columns: "userid", "type", and "value"
- Create a new UDC
- Navigate to your User Directory Connector in the Qlik Sense Management Console
- Create a new connection: Excel (via ODBC)
- Untick the checkbox Sync user data for existing users
- User table Name must match your first sheet ("[MyUsers$]" here)
- Attribute table name must match the second sheet ("[Attributes$]" here)
- Modify the connection string to point to your Excel file: DRIVER={Microsoft Excel Driver (*.xls, *.xlsx, *.xlsm, *.xlsb)};DBQ=C:\temp\SomeUsers.xls
- Validate and check in the logs
The following should be logged:
Connection creation verified.
Connection open verified.
Tables verified.
The userid field of the [MyUsers$] table is verified successfully.
The name field of the [MyUsers$] table is verified successfully.
The userid field of the [Attributes$] table is verified successfully.
The type field of the [Attributes$] table is verified successfully.
The value field of the [Attributes$] table is verified successfully. - Sync the UDC and check the logs
Started synchronizing all users for ...
Started syncing users
ODBC dataReader have been successfully created
Retrieved 3 entities from directory 'TEST' of type Repository.UserDirectoryConnectors.ODBC.OdbcExcel
Database done with 3 users and 0 groups in user directory (TEST, ...)
Ended saving users in repository
Finished synchronizing all users for ... - Verify that the users were added in the Qlik Sense Management Console
- Verify the attributes are reflected in the user's properties
Troubleshooting
The following errors you may be logged in C:\ProgramData\Qlik\Sense\Log\Repository\Trace\<SERVER>_UserManagement_Repository.txt
ERROR [IM006] [Microsoft][ODBC Driver Manager] Driver's SQLSetConnectAttr failed??ERROR [01S00] [Microsoft][ODBC Microsoft Access Driver]Invalid connection string attributeThis indicates the ODBC drivers are not correctly installed or an error in the connection string.ERROR [HY000] [Microsoft][ODBC Excel Driver]General error Unable to open registry key Temporary (volatile) Ace DSN for process 0x19e0 Thread 0x8f04 DBC 0xcf6bffe8Check the security permission for the XLS file; the service account must be granted READ. May also indicate an error in the connection string.Exception when trying to access the name field of the [MyUsers$]
Exception when trying to access the userid field of the [MyUsers$]
ERROR [07002] [Microsoft][ODBC Excel Driver] Too few parameters. Expected 1.??Exception when trying to access the name field of the [MyUsers$] table:
Verify that the name and userid columns in the file are correctly named and have not been renamed.
Environment
- Any version of Qlik Sense
- Verify if the drivers are already installed on your server:
-
The new Straight Table and old Table in Qlik Analytics
The new Straight Table was moved into the new native section of charts from the visualization bundle. The new Straight Table offers many improvements ... Show MoreThe new Straight Table was moved into the new native section of charts from the visualization bundle. The new Straight Table offers many improvements from the old table, and we encourage everyone to start using the new table instead of the old one.
This article aims to answer any frequently asked questions around this switch, beginning with:
- The old table will not be removed anytime soon. It will continue to work until further notice. We encourage you to subscribe to the Qlik Support Blog and What's New in Qlik Cloud to stay ahead of updates.
- The old table will not be updated further. Use the new table to benefit from future improvements.
- We monitor your feedback and will improve the functionality of the new table.
FAQ
What improvements does the Straight table come with?
Here are some examples:
- Grid styling
- Totals styling
- Pagination or virtual scroll
- Scrollbars outside of the table
- Add, delete many fields at the same time
- Cell font family, size and color styling
- Font styling by expression
- Column width options pixels, percentages
- Chart exploration, end user can pick dimensions and measures
- Header on/off
- Zebra striping
- Null value styling
- Selection info in export
- Totals in export
- Titles in export
- Cyclic dimension controls in dropdown
Will the old table be removed?
No, the old table will continue to work in the foreseeable future. Any changes will be notified in advance. Regardless, we highly recommend upgrading your tables as soon as possible to enjoy the new Straight Table's new functions.
How do I convert to the new table?
The easiest way to convert your tables is to drop the new table chart onto the old one.
- First, copy the old table
- Edit the sheet that includes the Table
- Open the Charts pane
- Drag the Straight table over your table
- Choose Convert to: Straight table
The new table looks different and offers more functionality that must be enabled to be used.
When will the old table be deprecated?
Not anytime soon, it will be a soft fade out. The new Straight Table will be the preferred choice for all new applications, and we anticipate many will upgrade to benefit from new functionality. Over time, the usage of the old table will diminish until most applications are using the new table anyway.
Will this affect Qlik Sense Enterprise on Windows?
Qlik Sense Enterprise on Windows will have the table feature aligned with Qlik Cloud Analytics in a future release.
I’m not happy with X and Y of the new table, will that be changed?
We’re closely monitoring feedback on the new table and are dedicated to creating the best possible experience, including improvements to accessibility. We are committed to accessible standards so people with disabilities can use our products. Printing, the tables should, of course, print in as consistent a manner as possible. As for usability, we aim to enable experienced users to reach functionality quickly, while also not overwhelming new users.
Originally, the old table was identified as table (retired). We removed the (retired) label.
Environment
- Qlik Analytics
-
Qlik Replicate: Test connection and other UI endpoint operations fails for Snowf...
After upgrading Qlik Replicate from 2023.11 to 2024.11 or 2025.5, testing connections and other UI endpoint operations fail whenever you are using an ... Show MoreAfter upgrading Qlik Replicate from 2023.11 to 2024.11 or 2025.5, testing connections and other UI endpoint operations fail whenever you are using an ADDON (such as UDF) that retrieves the password from the Vault.
The task works correctly. It is only the connection test that fails.
The following messages are from the task logs:
Error: SYS-E-HTTPFAIL, SYS-E-HTTPFAIL, Failed in prepare imp for Snowflake.. SYS,GENERAL_EXCEPTION,SYS-E-HTTPFAIL, Failed in prepare imp for Snowflake.,SYS,GENERAL_EXCEPTION,Failed in prepare imp for Snowflake,Failed to deobfuscate password
[INFRASTRUCTURE ]W: Password provider is not registered (at_secure.c:2454) (child_proc_exec.c:36)
[SERVER ]E: Failed to deobfuscate password [1003200] (cloud_imp.c:3700) (child_proc_exec.c:36)
[INFRASTRUCTURE ]T: Process id 396 : 00005244: 2025-06-26T12:42:26 [SERVER ]E: Failed in prepare imp for Snowflake [1003200] (cloud_imp.c:4770) (child_proc_exec.c:36)Resolution
This has been identified as a defect (ID RECOB-10010).
Fix Version
The fix is scheduled for the Qilk Replicate release in November 2025. See the Release Notes for details.
Cause
The child REPCTL process does not identify the ADDON.
Internal Investigation ID(s)
RECOB-10010
Environment
- Qlik Replicate
-
Qlik Replicate: Failure in resolving table name for objid %
A Qlik Replicate task with MSSQL as a source and Oracle as a target fails during Full Load and CDC. The error logged: Failure in resolving table name ... Show MoreA Qlik Replicate task with MSSQL as a source and Oracle as a target fails during Full Load and CDC.
The error logged:
Failure in resolving table name for objid %
Resolution
Failure in resolving table name for objid is commonly linked to temporary tables. When applications create temporary tables, they are logged in the transaction log. Qlik Replicate attempts to resolve the object ID from the log, but may fail if the object is unknown or transient.
To investigate further and to identify if there is data loss:
-
Run the following query to identify the table name:
SELECT name, object_id FROM sys.objects WHERE type = 'U' AND object_id LIKE '%' -- Replace % with the actual objid - Consult with your database administrator to determine whether the object is a temporary table or if any maintenance activities have been performed.
- If the table is a temporary table, you can disregard the error.
- If data loss occurs due to maintenance activities, please open a support case with Qlik Support.
Cause
This error is often associated with temporary tables. When an application creates temporary tables, they are written to the transaction log.
Qlik Replicate then attempts to read an unknown object ID from this log and fails to resolve it to a table name.
Environment
- Qlik Replicate
-
-
Qlik Sense Natural Language Query features
Insight Advisor supports natural language searches, such as Show me Product Inventory for Japan under 2500. The feature is available in Qlik Sense Ent... Show MoreInsight Advisor supports natural language searches, such as Show me Product Inventory for Japan under 2500. The feature is available in Qlik Sense Enterprise on Windows (September 2019 and later) and Qlik Cloud.
For Insight Advisor best practices with Qlik Cloud, see:
Best practices for improving performance of apps
Best practices for using business logic
Best practices for asking questions in Insight AdvisorFor general advice and information for Qlik Sense Enterprise on Windows (client managed), see the whitepaper attached to this article.
If you're instead looking for information about Qlik Insights Advisor chat, see Configuring Qlik Insight Advisor Chat in Qlik Sense Enterprise on Windows.
Related Content:
Creating visualizations with Insight Advisor
Using natural language with Insight Advisor
Tagging master items
Configuring Qlik Insight Advisor Chat in Qlik Sense Enterprise on Windows
Customizing logical models for Insight Advisor
-
Qlik Talend Product: Error 400 - Invalid SNI error after Installed Talend Runtim...
You may encounter an error : 400 - Invalid SNI when calling Talend Runtime API (Job as service) after installed 2025-02 patch or later. In the past be... Show MoreYou may encounter an error : 400 - Invalid SNI when calling Talend Runtime API (Job as service) after installed 2025-02 patch or later. In the past before the patch version R2025-02 of Talend Runtime Server, it did work well when using the same certificate for SSL connection with Talend Runtime Server and did not cause any issue.
The SNI validation is active after 2025-02 patch or later.
Resolution
There are three options to slove this issue
- Obtain and install a proper certificate that references the correct host name and then access it with the hostname rather than by IP.
- Disable SNI host check.
- Tell Talend component to resolve IP as hostname
Disable SNI Host Check
This has the same security risk as jetty before it was updated (low security)
In <RuntimeInstallationFolder>/etc/org.ops4j.pax.web.cfg file, please add
jetty.ssl.sniRequired=false
and
jetty.ssl.sniHostCheck=false
Or configuring these jetty parameters in <RuntimeInstallationFolder>/etc/jetty.xml or jetty-ssl.xml file
- Find the <New class="org.eclipse.jetty.server.SecureRequestCustomizer"> block in your jetty.xml or jetty-ssl.xml
- Edit the <Arg name="sniRequired" ...> and <Arg name="sniHostCheck" ...> lines so that the properties' defaults are set to false as shown below:
<New id="sslHttpConfig" class="org.eclipse.jetty.server.HttpConfiguration">
<Arg><Ref refid="httpConfig"/></Arg>
<Call name="addCustomizer">
<Arg>
<New class="org.eclipse.jetty.server.SecureRequestCustomizer">
<Arg name="sniRequired" type="boolean">
<Property name="jetty.ssl.sniRequired" default="false"/>
</Arg>
<Arg name="sniHostCheck" type="boolean">
<Property name="jetty.ssl.sniHostCheck" default="false"/>
</Arg>
<Arg name="stsMaxAgeSeconds" type="int">
<Property name="jetty.ssl.stsMaxAgeSeconds" default="-1"/>
</Arg>
<Arg name="stsIncludeSubdomains" type="boolean">
<Property name="jetty.ssl.stsIncludeSubdomains" default="false"/>
</Arg>
</New>
</Arg>
</Call>
</New>
Resolve IP to Hostname
If the certification includes the domain name, you should use that domain name instead of the IP with the Jetty security updates in Talend Runtime Server.
But if your DNS server does not resolve the IP, you must call it by the IP address, so please check it at first to see if the workaround is feasible for your current situation.
In the examples the hostname is unresolvedhost.net and the IP is 10.20.30.40.
Try this API call at the command line:
curl -k -X GET --resolve unresolvedhost.net:9001:10.20.30.40 https://unresolvedhost.net:9001/services/
or
curl -k -X GET -H "Host: unresolvedhost.net" https://10.20.30.20:9001/services/
If this works, in your Talend component that makes the API call, go to "Advanced settings" or "Headers" table, add a row with Key: Host and Value: The hostname that matches your SSL certificate (e.g. unresolvedhost.net)
This will instruct Talend to send the correct Host header, which most HTTP clients (including Java's HttpClient) will also use as the SNI value during the TLS handshake.
Cause
The SNI enforcement is there for a security reason. With the 2025-02 patch, the Jetty components on Talend Runtime Server resolved a CVE security issue where they allowed a hostname to connect to a server that doesn't match the hostname in the server's TLS certificate.
Certificates require the URI not to be localhost or an IP address, and to have at least one dot, so a fully qualified domain name is best.
Related Content
Environment
-
Binary load fails with General Script Error when using app binary instead of .qv...
A binary load command that refers to the app ID (example Binary[idapp];) does not work and fails with: General Script Error or Binary load fails with ... Show MoreA binary load command that refers to the app ID (example Binary[idapp];) does not work and fails with:
General Script Error
or
Binary load fails with error Cannot open file
Before Qlik Sense Enterprise on Windows November 2024 Patch 8, the Qlik Engine permitted an unsupported and insecure method of binary loading from applications managed by Qlik Sense Enterprise on Windows.
Due to security hardening, this unsupported and insecure action is now denied.
Binary loads of Qlik Sense applications require a QVF file extension. In practice, this will require exporting the Qlik Sense app from the Qlik Sense Enterprise on Windows site to a folder location from which a binary load can be performed. See Binary Load and Limitations for details.
Example of a valid binary load:
Binary [lib://My_Extract_Apps/Sales_Model.qvf];Example of an invalid binary load:
"Binary [lib://Apps/777a0a66-555x-8888-xx7e-64442fa4xxx44];"Environment
- Qlik Sense Enterprise on Windows November 2024 Patch 8 and any later releases
-
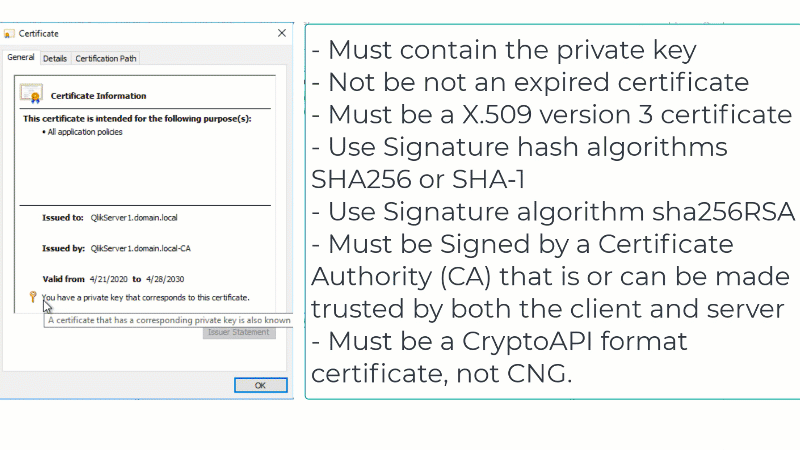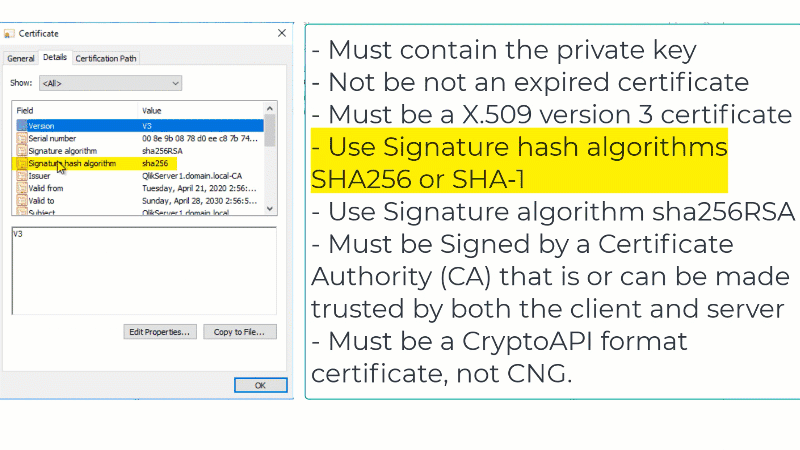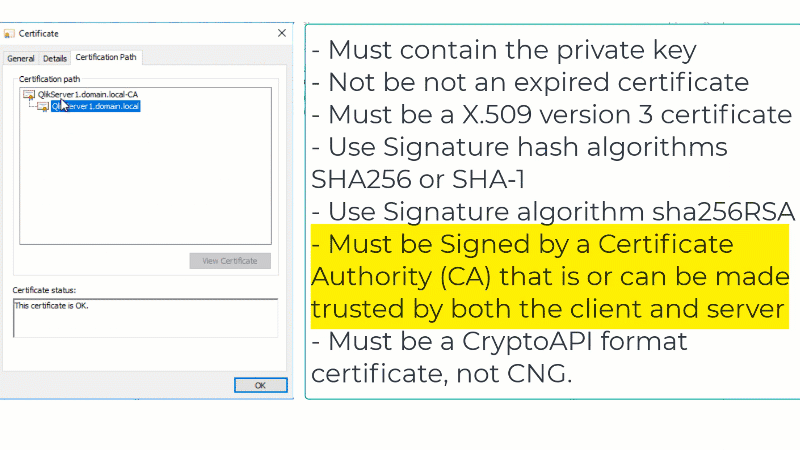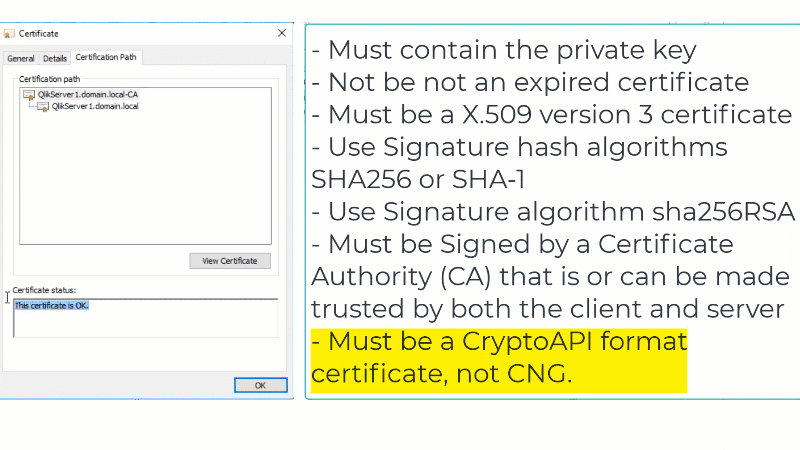
Qlik Sense Enterprise on Windows: Compatibility information for third-party SSL ...
A third-party certificate was configured in the Qlik Sense Proxy, but is not being used. The connection is not private" NET::ERR_CERT_COMMON_NAME_INVA... Show MoreA third-party certificate was configured in the Qlik Sense Proxy, but is not being used.
The connection is not private" NET::ERR_CERT_COMMON_NAME_INVALID may be displayed on HUB access.
Qlik Sense Enterprise on Windows uses self-signed and self-generated certificates to protect communication between services, as well as user web traffic to the hub and management console. It is possible to use a third-party-issued SSL certificate to protect client web traffic. Using the self-signed certificate will cause a certificate warning to be displayed in the web browser (such as Google Chrome or Internet Explorer).
If the third-party certificate for the Qlik Sense Proxy Service is not fully compatible with Qlik Sense or it does not have the correct attributes and cyphers, the Qlik Sense Repository Service will revert to using the default certificates. The following error may occur in the Proxy Security logs:
Example: C:\ProgramData\Qlik\Sense\Log\Proxy\Trace\HOSTNAME_Security_Proxy.txtNo private key found for certificate 'CN=qliksense.domain.com' ([CERTIFICATE THUMBPRINT HERE]) Couldn't find a valid ssl certificate with thumbprint [CERTIFICATE THUMBPRINT HERE] Reverting to default Qlik Sense SSLCertificate Set certificate 'CN=qliksenseserver1.domain.com' ([CERTIFICATE THUMBPRINT HERE]) as SSL certificate presented to browser
Resolution:
In order for Qlik Sense Enterprise to correctly recognize the third-party certificate as valid, the certificate will have to meet the following requirements:
Note: Root and Intermediate CA certificates need to be correctly installed. Should any be missing, Qlik Sense proxy will not use the server certificate and will revert back to using the self-signed certificate instead.
Certificates that are known to work well with Qlik Sense have the following attributes:- Certificates that are x509 version 3. More information including filename extensions under https://en.wikipedia.org/wiki/X.509
- Use signature algorithm sha256RSA
- Use signature hash algorithm sha256
- Signed by a valid, and OS/browser configured , CA
- Are valid according to date restrictions (valid from/valid to)
- Key in format CryptoAPI (not in CNG)
- The certificate itself has to contain private key no matter what Qlik Sense version.
Related Content:
How to: Change the certificate used by the Qlik Sense Proxy to a custom third party certificate
-
How to disable 'Base memory size' info and 'StaticByteSize' calls in Qlik Sens E...
After upgrading to Qlik Sense Enterprise on Windows May 2023 (or later), the Qlik Sense Repository Service may cause CPU usage spikes on the central n... Show MoreAfter upgrading to Qlik Sense Enterprise on Windows May 2023 (or later), the Qlik Sense Repository Service may cause CPU usage spikes on the central node. In addition, the central Engine node may show an increased average RAM consumption while a high volume of reloads is being executed.
The Qlik Sense System_Repository log file will read:
"API call to Engine service was successful: The retrieved 'StaticByteSize' value for app 'App-GUID' is: 'Size in bytes' bytes."
Default location of log: C:\ProgramData\Qlik\Sense\Log\Repository\Trace\[Server_Name]_System_Repository.txt
This activity is associated with the ability to see the Base memory size in the Qlik Sense Enterprise Management Console. See Show base memory size of apps in QMC.
Resolution
The feature to see Base memory size can be disabled. This may require an upgrade and will require downtime as configuration files need to be changed.
Take a backup of your environment before proceeding.
- Verify the version you are using and verify that you are at least on one of the versions (and patches) listed:
- May 2023 Patch 13
- August 2023 Patch 10
- November 2023 Patch 4
- February 2024 IR
- Back up the following files:
- C:\Program Files\Qlik\Sense\CapabilityService\capabilities.json
- C:\Program Files\Qlik\Sense\Repository\Repository.exe.config
*where C:\Program Files can be replaced by your custom installation path.
- Stop the Qlik Sense Repository Service and the Qlik Sense Dispatcher Service
- Open and edit the capabilities.json with a text editor (using elevated permissions)
- Open C:\Program Files\Qlik\Sense\CapabilityService\capabilities.json
- Locate: "enabled": true,"flag": "QMCShowAppSize"
- Change this to: "enabled": false,"flag": "QMCShowAppSize"
- Save the file
- Open and edit the repository.exe.config with a text editor (using elevated permissions)
- Open C:\Program Files\Qlik\Sense\Repository\Repository.exe.config
- Locate: </appSettings>
- Before the </appSettings> closing statement, add:
<add key="AppStaticByteSizeUpdate.Enable" value="false"/> - Save
- Start all Qlik Sense services
- Verify you can access the Management Console
If any issues occur, revert the changes by restoring the backed up files and open a ticket with Support providing the changed versions of repository.exe.config, capabilities.json mentioning this article.
Related Content
Show base memory size of apps in QMC
Internal Investigation:
QB-22795
QB-24301Environment
Qlik Sense Enterprise on Windows May 2023, August 2023, November 2023, February 2024.
- Verify the version you are using and verify that you are at least on one of the versions (and patches) listed:
-
How to create NPrinting GET and POST REST connections
NPrinting has a library of APIs that can be used to customize many native NPrinting functions outside the NPrinting Web Console. Environment: Qlik N... Show MoreNPrinting has a library of APIs that can be used to customize many native NPrinting functions outside the NPrinting Web Console.
Environment:
An example of two of the more common capabilities available via NPrinting APIs are as follows
- Connection reloads
- Publish Task executions
These and many other public NPrinting APIs can be found here: Qlik NPrinting API
In the Qlik Sense data load editor of your Qlik Sense app, two REST connections are required (These two REST Connectors must also be configured in the QlikView Desktop application>load where the API's are used. See Nprinting Rest API Connection through QlikView desktop)
- GET
- POST
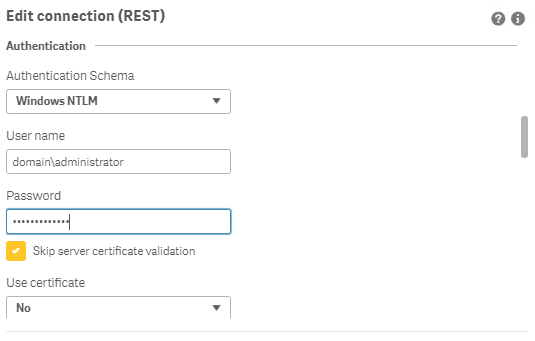
Requirements of REST user account:
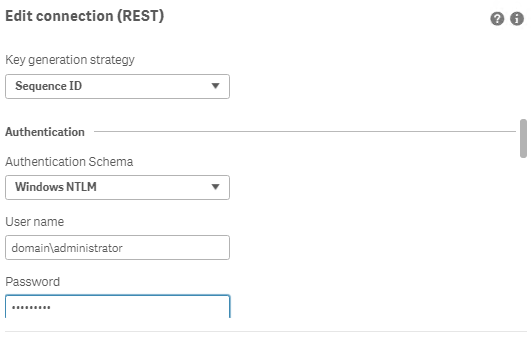
- Windows Authentication is required in both these connectors. The required user account is the NPrinting service account (which is also ROOTADMIN on the Qlik Sense server)
- This user account must also be a member of the NPrinting 'Administrators' Security Role on the NPrinting Server.
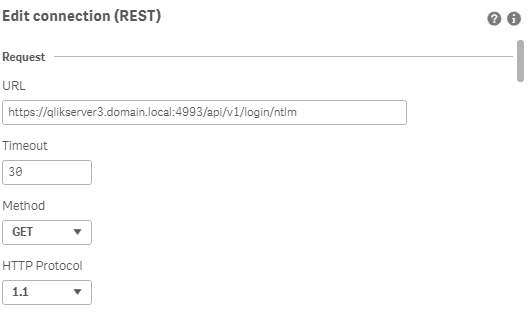
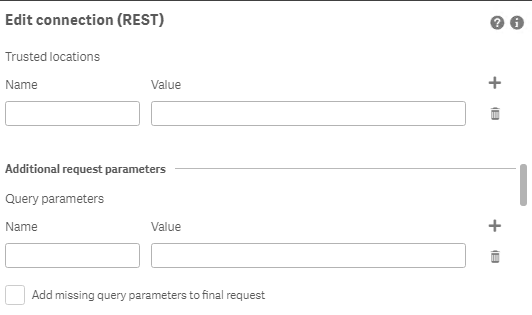
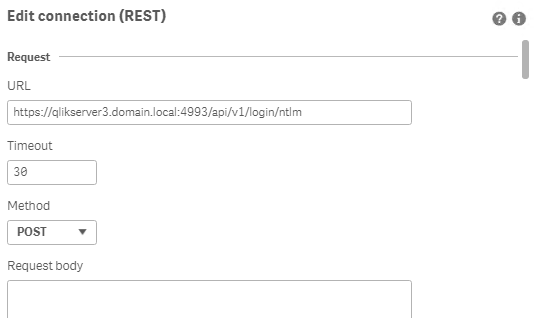
Creating REST "GET" connections
Note: Replace QlikServer3.domain.local with the name and port of your NPrinting Server
NOTE: replace domain\administrator with the domain and user name of your NPrinting service user account
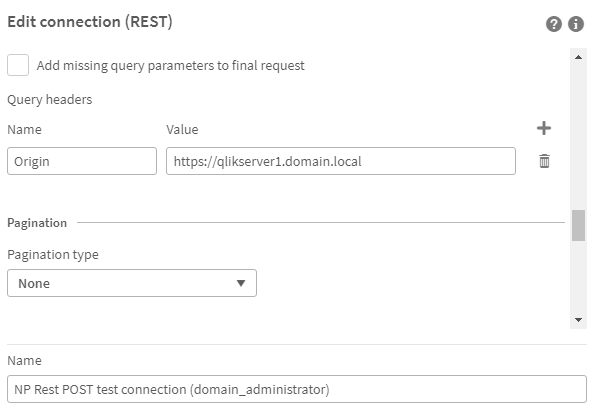
Creating REST "POST" connections
Note: Replace QlikServer3.domain.local with the name and port of your NPrinting Server
NOTE: replace domain\administrator with the domain and user name of your NPrinting service user account
Ensure to enter the 'Name' Origin and 'Value' of the Qlik Sense (or QlikView) server address in your POST REST connection only.
Replace https://qlikserver1.domain.local with your Qlik sense (or QlikView) server address.
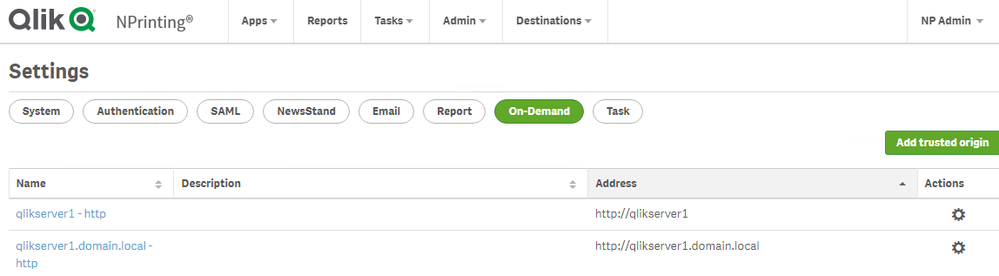
Ensure that the 'Origin' Qlik Sense or QlikView server is added as a 'Trusted Origin' on the NPrinting Server computer
Related Content
- Distribute NPrinting reports after reloading a Qlik App
- Extending Qlik NPrinting
- Run a Qlik NPrinting API POST command via QlikView reload script
- Troubleshooting Common NPrinting API Errors
NOTE: The information in this article is provided as-is and to be used at own discretion. NPrinting API usage requires developer expertise and usage therein is significant customization outside the turnkey NPrinting Web Console functionality. Depending on tool(s) used, customization(s), and/or other factors ongoing, support on the solution below may not be provided by Qlik Support.
-
Qlik Talend Studio R2025-06v3 Fails to Return Correct Value for LAST_QUERY_ID() ...
When upgrading to Qlik Talend Studio R2025-06v3 or later tSnowflakeRow with Snowflake-jdbc-driver-3.22.0 that uses the function LAST_QUERY_ID() in com... Show MoreWhen upgrading to Qlik Talend Studio R2025-06v3 or later tSnowflakeRow with Snowflake-jdbc-driver-3.22.0 that uses the function LAST_QUERY_ID() in command always returns “Alter session set JDBC_USE_SESSION_TIMEZONE=false” instead of the the correct query id(query id last query ran)as a result.
Resolution
To have LAST_QUERY_ID() return the last query and not the alter session query, it is required to modify the command to use LAST_QUERY_ID(-2).
For example:
Select query used in tSnowflakeRow component
"SELECT LAST_QUERY_ID() as LAST_QUERY_ID(-2);"
Cause
In Studio R2024-04, a new checkbox "Use Session Timezone" was added to the tSnowflakeInput and tSnowflakeRow components. The default value is unchecked (Alter session set JDBC_USE_SESSION_TIMEZONE=false) to avoid Snowflake regression issue Incorrect timezone handling for java.sql.Time, However this does bring the side effect of snowflake functions like LAST_QUERY_ID() returning the wrong value.
Related Content
2025-04-studio-known-issues for 'Use Session Timezone' option in the Snowflake components
Environment